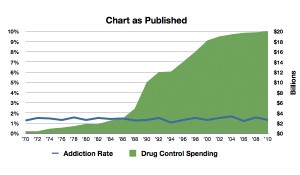
Fig. 1: “A Chart That Says the War on Drugs
Isn’t Working,” as published, with two y-axes.
(Click each image for a larger view.)

By any measure, the War on Drugs has been and continues to be a failure. Although an ever-increasing number of political figures is willing to admit this, the argument has not been presented to the public as clearly as it might.

Recently, for example, salon.com drew attention to a chart reproduced in theatlanticwire.com.  The graphic (Fig. 1), which I’ve recreated here for easier comparison*, purports to show how the 40-year-old “war” has been, according to salon.com, “an extremely expensive Huge Government boondoggle.” While annual federal spending to “control” drugs has increased 20-fold during that period, the national rate of addiction has remained largely unchanged at between 1% and 2% of the U.S. population.

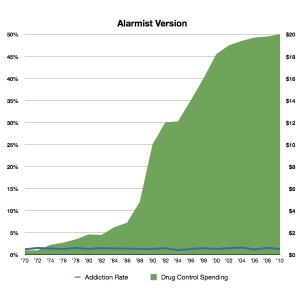
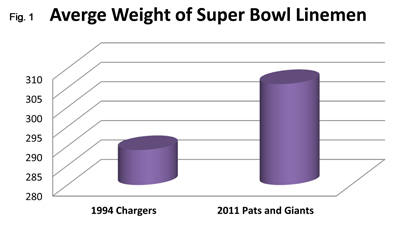
Fig. 2: This chart exaggerates the disparity between the two sets of data and gives the impression of an even greater degree of waste.

Figure 1 shows that relationship, sort of, but it misleads the viewer. How? By distorting the relationship between the two sets of numbers, using different units of measurement. Instead of comparing percentages to percentages or dollars to dollars, the published chart “compares” percentages to dollars, each according to its own arbitrary scale, as shown on the left and right y-axes, respectively.

To see how pernicious a practice this is, consider how manipulating the chart’s two y-axes scales can reinforce contradictory conclusions.

An “Alarmist Version” of the original chart (Fig. 2) heightens the suggestion that the War on Drugs has been tremendously wasteful.

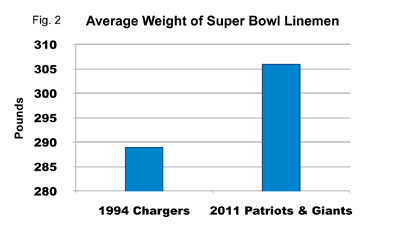
Fig. 3: This chart minimizes the relationship between the two sets of data, suggesting ineffectiveness perhaps, but negligible waste.

A “Dismissive Version” (Fig. 3), on the other hand, suggests that the War on Drugs has been hardly wasteful at all.

I’m sure the original chart was designed in good faith. The fact remains, however, that it distorts the data it supposedly illustrates, a inherent flaw of charts with two y-axes scales. What makes the published chart especially disappointing is that it carelessly assumes that its depiction is value-free.

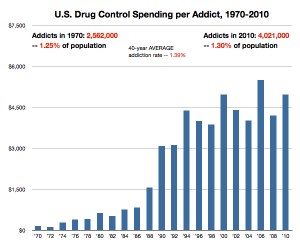
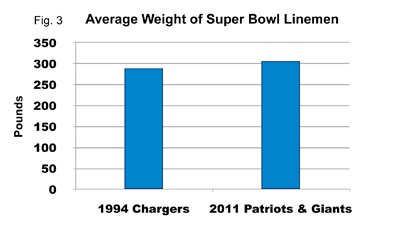
Fig. 4: This chart shows a large increase in control dollars spent per addict over a time when the addiction rate remained essentially flat.

What would be a better way of expressing these facts, one without bias (inasmuch as this is possible)?

Consider “U.S. Drug Control Spending per Addict, 1970-2010” (Fig. 4). By converting the two sets of data to a common unit of measurement, control dollars spent per addict, this chart more accuratetly shows the growth in spending during a time when the rate of addiction remained roughly constant. Not as dramatic as the preceding versions certainly, but considerably more honest.

Can you design a different chart to show this information with minimal bias? What dual y-axes charts have you seen lately?
 
 
 
* Figures 1-4 are based on a close reading of the original theatlanticwire.com chart instead of the actual numbers, which weren’t readily available. Theatlanticwire.com identifies the sources as the U.S. Department of Health and Human Services and the International Centre for Science in Drug Policy by way of documentary filmmaker Matt Groff.

Return

Statistical graphics are commonly used to “bulk up” news stories and add a sense of scientific authority to their claims. However, most charts in the popular media are junk–hard to read and misleading at the same time. This one (fig. 1), for example, appeared widely after the suicide of former San Diego Chargers linebacker Junior Seau.

The tragedy prompted speculation about how a career’s worth of head injuries caused by collisions against today’s larger opponents might’ve contributed to Seau’s death. But the chart is awful. What are the two average weights supposed to be–286 or 295? 303 or 310?

The first step to improving this chart (after fixing the typo in the title) is to dump the fake 3-D effect (fig. 2). Now you can clearly see that the correct values are 289 and 306.

The 2-D chart is still deceiving, however, because it suggests that the average 2011 player was nearly three times the size of the average 1994 player.

Fixing this is simply a matter of basing the chart’s scale on zero (fig. 3). This makes it obvious that the average 2011 player was roughly 6% bigger than his 1994 counterpart.

This redesigned chart is now accurate and easy to read, which should be the absolute minimum standard for statistical graphics. It’s still a bit dry, but any attempt to “prettify” the numbers should be careful to maintain this standard. Here’s what an accurate, legible, more visually interesting statistical graphic might look like–don’t you agree?